Assignment 9:
Peddling Pedal Triangles
by
Kristina Little
Assignment 9:
Peddling Pedal Triangles
by
Kristina Little
A pedal triangle is a triangle whose vertices are the feet of the perpendiculars from any given point P to the sides of the triangle.
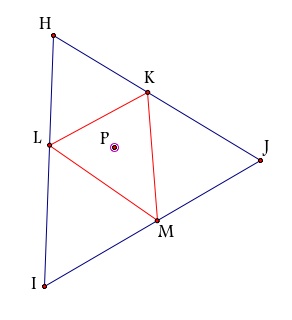
A special type of pedal triangle is the medial triangle which is formed by joining the midpoints of the sides of the triangle. Interestingly enough, the circumcircle of the medial triangle is the nine point circle.
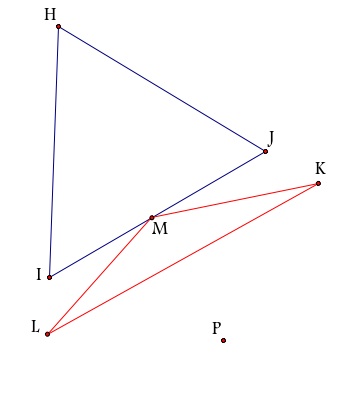
The pedal point is allowed to be inside or outside the triangle. In the following script tool, drag the pedal point inside and outside of the triangle. Also, when the pedal point lies on the cirumcircle of the given triangle the pedal triangle degenerates into a line. Look for this special case as well.
Here is a GSP script tool to help you create and react to any given triangle and any given pedal point. Watch how the pedal triangle changes in relation to moving the pedal point around. Do you see the special triangles that the introduction mentioned above? Try using this script tool in tandem with the other triangle script tools from Assignment 5: Lost Art of Scripting.
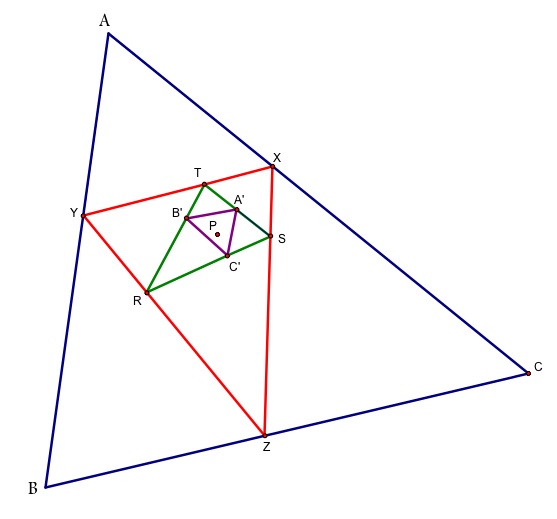
Now we will look at the above special construction of a pedal triangle. Here we have constructed the pedal triangle of the pedal triangle of the pedal triangle of the original triangle. We are led to believe that this third pedal triangle is similar to the original triangle. Do we have enough information to prove this?
Through construction we can show that pedal point P lies on the circumcircles of triangles AXY, TXS, C'A'S, TRY, and C'RB'. If you would like to see this, simply construct through each of the triangles listed above with its corresponding circumcircle. You may also use the GSP scripts to simply reiterate this construction from Assignment 5: Lost Art of Scripting.
Circumcircles of the necessary triangles. Please feel free to move the vertices of the original triangle to see in greater detail the properties of the circumcircles.
From previous geometry knowledge, we know that the circumcircle of a triangle has this property when applied to the angles of the triangle.
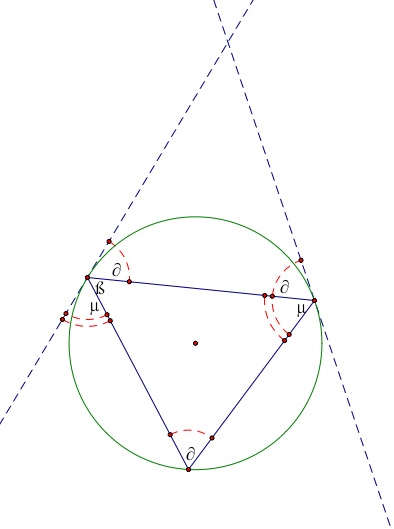
Therefore we can now show that 
.
(Angles listed above are marked in color.)
And also 
.
(Angles listed above are marked in color.)
In conclusion of showing these angles are all congruent, because we know segment AP divides angle BAC in some way, there are equal counterparts in angle ZXY and ZYX, again in angle TSR and TRS, and finally both parts at angle A'C'B'. We can conclude triangle ABC and triangle A'B'C' have equal angles at angle BAC and A'C'B'. Similarly, they have equal angles at angle ABC and angle B'A'C'.
Further, this theorem has been generalized for n-sided polygons stated such, the nth pedal n-gon is similar to the original one. Do you believe that the proof for this generalization has similarities to our proof for the triangle? Why?